property>volume
What is Volume?
Simply put, the volume of any object, either one observed in nature or theoretical, is the space that it occupies in three dimensions. This space is measured in units of cubic length; the SI unit being cubic meters. Lines, points, or two dimensional objects are described as having zero volume because they do not occupy three dimensions.
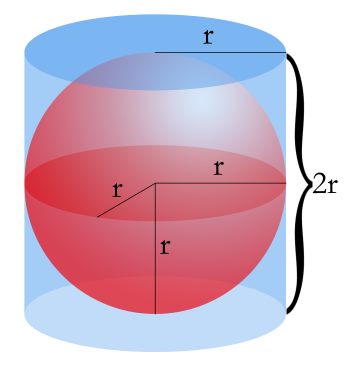
There are many ways to calculate the volumes of various three dimensional shapes. The simplest example is the sphere which has a volume of (4/3).π.r3 where r is the radius of the sphere. The volume of a sphere can be considered as 2/3 of the volume of the smallest cylinder that contains the sphere (where h = 2r).
Other examples include the cube, which is simply the length of one of its sides cubed. Here are some formulae for some common geometric shapes:
Cylinder: V = π.r2.h
where r is the radius of a cross-sectional circular slice of the cylinder and h is its height.
Pyramid: V = B.H/3
where B is the length of the base, and H is the height of the pyramid.
Calculus provides many of the tools used to derive the different formulae for volume. The integral of the cross-sectional areas of a three-dimensional figure taken with respect to the height of that figure (also known as the axis perpendicular to the sections, or the dimension along which the sections are “stacked”) will yield the volume of the shape. To put this into a formal description, the following is true:
V = ∫ A(h) dh
where A(h) is a function defining some kind of infinitesimal cross sectional area with respect to the location of that cross sectional area on the axis h. Of course with calculus one can conceive many different ways to integrate over a shape, and the ease or difficulty of the integral will depend on this. For example, it is known that it is easier to use an integral to derive the volume of a sphere by integrating the surface area of the sphere with respect to the radius of the sphere. This approach works because one is effectively adding up the all of the infinitesimal radii that go from the centre of the sphere to its outer surface.